7.2. Integration Techniques
Theorem 7.2.8: Mean Value Theorem for Integration
If f and g are continuous functions defined on
[a, b] so that g(x) 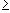 0,
then there exists a number c
0,
then there exists a number c 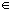 [a, b]
with
[a, b]
with
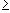 0,
then there exists a number c
0,
then there exists a number c 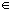 [a, b]
with
[a, b]
with
f(x) g(x) dx = f(c)
g(x) dx
Proof
Define the numbersm = inf{ f(x): xThen we have m[a, b] }
M = sup{ f(x): x[a, b] }
 f(x)
f(x)  M
and since g is non-negative we also have
M
and since g is non-negative we also have
m g(x)By the properties of the Riemann integral this implies thatf(x) g(x)
M g(x)
mTherefore there exists a number d between m and M such thatg(x) dx

f(x) g(x) dx
M
g(x) dx
dBut since f is continuous on [a, b] and d is between m and M, we can apply the Intermediate Value Theorem to find a number c such that f(c) = d. Theng(x) dx =
f(x) g(x) dx
f(c)which is what we wanted to prove.g(x) dx =
f(x) g(x) dx
 Interactive Real Analysis
Interactive Real Analysis