8.3. Series and Power Series
Example 8.3.4 (a): Geometric Series Function
Define
fn(x) = xn for
x 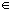 [-r, r], where
0 < r < 1. Then the function
[-r, r], where
0 < r < 1. Then the function
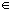 [-r, r], where
0 < r < 1. Then the function
[-r, r], where
0 < r < 1. Then the function
f(x) =is continuous on [-r, r]. Can you find a simpler expression for f?fn(x) =
xn
If
-1 < -r  x
x  r < then
|| xn ||[-r, r] = rn. Since
r < then
|| xn ||[-r, r] = rn. Since
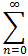 rn <
rn <
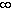 the Weierstrass convergence
theorem applies immediately to show that the series represents a continuous
function.
the Weierstrass convergence
theorem applies immediately to show that the series represents a continuous
function.
Of course we have seen this series before and know it as geometric series with limiting function 1/1-x. But this time we know as an application of Weierstrass' theorem that the series is a continuous function, whether it has a simpler representation or not.
 Interactive Real Analysis
Interactive Real Analysis