1.4. Natural Numbers, Integers, and Rational Numbers
Theorem 1.4.4: The Rationals
Let A be the set N x N - {0} and define a
relation r on N x N - {0} by saying that (a,
b) is related to (a', b') if a * b' = a' * b. Then this
relation is an equivalence relation.
If [(a, b)] and [(a', b')] denotes the equivalence classes containing (a, b) and (a', b'), respectively, and if we define the operations
- [(a, b)] + [(a', b')] = [(a * b' + a' * b, b * b')]
- [(a, b)] * [(a', b')] = [(a * a', b * b')]
Proof:
The proof is much similar to proving the similar statement regarding the integers. The details are left as an exercise.
Notice the requirement that
(a,b) 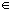 N x N - {0}
i.e. the integer b can not be zero. If we did allow both a
and b to become zero, the relation would not be an equivalence
relation any longer. As a hint: what pairs (a, b) would be related
to (0,0) ?
N x N - {0}
i.e. the integer b can not be zero. If we did allow both a
and b to become zero, the relation would not be an equivalence
relation any longer. As a hint: what pairs (a, b) would be related
to (0,0) ?
 Interactive Real Analysis
Interactive Real Analysis