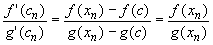Interactive Real Analysis

• Real Analysis
• 1. Sets and Relations
• 2. Infinity and Induction
• 3. Sequences of Numbers
• 4. Series of Numbers
• 5. Topology
• 6. Limits, Continuity, and Differentiation
• 6.1. Limits
• 6.2. Continuous Functions
• 6.3. Discontinuous Functions
• 6.4. Topology and Continuity
• 6.5. Differentiable Functions
• 6.6. A Function Primer
• 7. The Integral
• 8. Sequences of Functions
• 9. Historical Tidbits
• Java Tools

6.5. Differentiable Functions

Theorem 6.5.15: l Hospital Rules

If f and g are differentiable in a neighborhood of x = c, and f(c) = g(c) = 0, then

provided the limit on the right exists. The same result holds for one-sided limits.

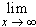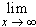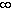If f and g are differentiable and f(x) = g(x) = - then

provided the last limit exists.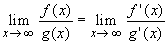

Context

Proof:

The first part can be proved easily, if the right hand limit equals f'(c) / g'(c): Since f(c) = g(c) = 0 we have

Taking the limit as x approaches c we get the first result. However, the actual result is somewhat more general, and we have to be slightly more careful. We will use a version of the Mean Value theorem:

Take any sequence {x_n} converging to c from above. All assumptions of the generalized Mean Value theorem are satisfied (check !) on [c, x_n]. Therefore, for each n there exists a number c_n in the interval (c, x_n) such that

Taking the limit as n approaches infinity will give the desired result for right-handed limits. The proof is similar for left handed limits and therefore for 'full' limits.

The proof of the last part of this theorem is left as an exercise.