6.6. A Function Primer
Countable Discontinuities
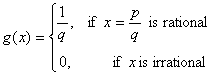
Proof:
The actual proof is left as an exercise. However, you may want to make use of the following fact:Lemma
If r = p / q is a rational number (in lowest terms) define a function with domain Q via f(r) = 1 / q. Then the limit of f(r) as r approaches any real number is zero.Proof of Lemma:
Take any sequence { rn } of rational numbers converging to a fixed number a (which could be rational or irrational). Since the sequence converges, it is bounded. For simplicity assume that all rational numbers rn are in the interval [0, K] for some integer K. Now take any > 0 and pick an integer M such that
1 / M <
> 0 and pick an integer M such that
1 / M <  . Because each rational number is the
quotient of two positive integers, we have:
. Because each rational number is the
quotient of two positive integers, we have:
- at most K of the rational numbers rn in the interval [0, N] can have denominator equal to 1
- at most 2 K of the rational numbers rn in the interval [0, N] can have denominator equal to 2
- ...
- at most M * K of the rational numbers rn in the interval [0, N] can have denominator equal to M
| f(rn) | < 1 / M <Sincefor all n > N
 > 0 was arbitrary, that
means that the limit of f(rn) must be zero, as needed. Our assumption
that the numbers rn were all positive can easily be dropped,
and a similar proof would work again.
> 0 was arbitrary, that
means that the limit of f(rn) must be zero, as needed. Our assumption
that the numbers rn were all positive can easily be dropped,
and a similar proof would work again.

Using this lemma it should not be too hard to prove the original assertion.
 Interactive Real Analysis
Interactive Real Analysis