5.2. Compact and Perfect Sets
Examples 5.2.7(b):
Can you find infinitely many closed sets such that their
intersection is empty and such that each set is contained in
its predecessor ? That is, can you find sets
A j such that
A j+1
 A j and
A j and
 A j= 0 ?
A j= 0 ?
It is easy to simply find some closed sets with empty intersection.
For example, the intersection of all intervals of the form
[n, n+1] is certainly empty.
 A j and
A j and
 A j= 0 ?
A j= 0 ?
To find sets contained in one another is slightly more complicated.
We might try sets of the form
A j = [0, 1 / j] for all j.
Then
A j+1
 A j,
but their intersection contains the point {0}.
A j,
but their intersection contains the point {0}.
Let
A j = [j, 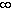 ).
Then
A j+1
).
Then
A j+1
 A j,
because [j + 1,
A j,
because [j + 1, 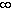 )
)
 [j,
[j, 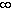 ).
But by the Archimedian principle, the intersection of all
sets Aj is empty.
).
But by the Archimedian principle, the intersection of all
sets Aj is empty.
 Interactive Real Analysis
Interactive Real Analysis