Ratio Test
Definition: Euler Series
Proof:
It is easy to show that this series converges. We could use, for example, the ratio test:Hence, the series converges by the ratio test. However, this test says nothing about the actual limit of the series. We have to be a lot more sophisticated to find the actual limit of this series.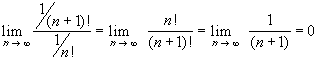
Recall that we have defined Euler's number as the limit of the Euler sequence. The proof that the above sum equals that limit is very similar to the proof that the Euler sequence converges in the first place.
First, we use the binomial theorem to expand the expression
From that expansion it is clear that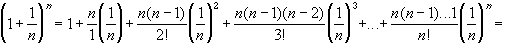
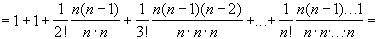
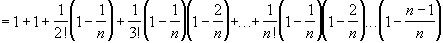
because each term in parenthesis is smaller than one. On the other hand, we also have: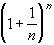

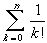
for N < n, because each term in parenthesis is greater than zero. But then, taking the limit as n approaches infinity, we have: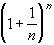
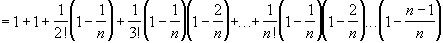
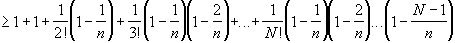
Hence, taking the limit as N approaches infinity, we have the sum squeezed in between the limit of Euler's sequence, which we know is equal to e. That proves the result.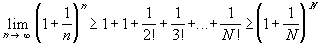

 Interactive Real Analysis
Interactive Real Analysis is called Euler's series. It converges to Euler's number
e.
is called Euler's series. It converges to Euler's number
e.